 class HCL_RnVector_d : public HCL_EuclideanVector_d
class HCL_RnVector_d : public HCL_EuclideanVector_d  class HCL_RnVector_d : public HCL_EuclideanVector_d
class HCL_RnVector_d : public HCL_EuclideanVector_d HCL_RnVector_d is a memory-based vector class representing vectors in Rn
| | Constructors
| ||||||||||
| | Data Access
|
Access to components.
Access to dimension
Array operations - ``Matlab'' methods.
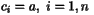
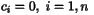
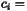 pseudorandom numbers uniformly distributed between -1 and 1
pseudorandom numbers uniformly distributed between -1 and 1
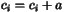 - overload of Add(...) with scalar argument a la Matlab
- overload of Add(...) with scalar argument a la Matlab
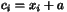 - overload of Add(...) with scalar argument a la Matlab
- overload of Add(...) with scalar argument a la Matlab
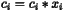 - matrix multiply by diag(x)
- matrix multiply by diag(x)
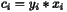
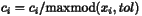 - matrix division by diag(x)
- matrix division by diag(x)
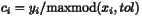 - matrix division by diag(x)
- matrix division by diag(x)
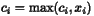
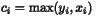
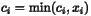
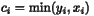
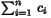
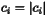
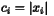
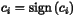
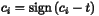
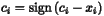
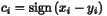
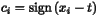
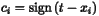
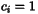 if
if 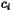 >
> 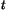 , else 0
, else 0
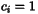 if
if 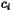 >
> 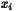 , else 0
, else 0
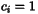 if
if 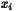 >
> 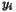 , else 0
, else 0
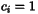 if
if 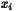 >
> 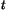 , else 0
, else 0
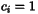 if
if 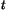 >
> 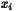 , else 0
, else 0
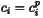 - arbitrary real power
- arbitrary real power
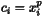 - arbitrary real power
- arbitrary real power
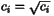 - componentwise square root
- componentwise square root
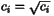 - componentwise square root
- componentwise square root
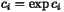
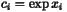
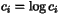 - componentwise natural log
- componentwise natural log
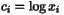 - componentwise natural log
- componentwise natural log
constructors and destructor
Subarray extraction.
Vector operations (z is *this, which is C++-ese for the instance of the class invoking the method)
HCL_RnVector_d is a memory-based vector class representing vectors in Rn. Vectors are stored as arrays, and most operations on the vectors take advantage of level-1 BLAS operations.
![]() HCL_RnVector_d( HCL_RnSpace_d * s )
HCL_RnVector_d( HCL_RnSpace_d * s )
![]() HCL_RnVector_d( int n, double *data = NULL )
HCL_RnVector_d( int n, double *data = NULL )
![]() HCL_RnVector_d( const HCL_RnSpace_d & s, char * fname = NULL )
HCL_RnVector_d( const HCL_RnSpace_d & s, char * fname = NULL )
![]() HCL_RnVector_d( char * fname, int save = 1 )
HCL_RnVector_d( char * fname, int save = 1 )
![]() void Save( char * fname )
void Save( char * fname )

this page has been generated automatically by doc++
(c)opyright by Malte Zöckler, Roland Wunderling
contact: doc++@zib.de